This tutorial discusses the solution of the classical Bretherton problem in which an air-finger is driven steadily into a 2D fluid-filled, rigid-walled channel.
The driver code listed below was originally developed as a test-bed for the paper
in which we studied the elastic-walled equivalent, paying particular attention to the effect of transverse gravity which plays quite an important role in this problem.
Compared to other tutorials, there is no detailed discussion of the driver code itself because the implementation is somewhat messy. However, given that the driver code is (of course!) very well documented you should be able to figure out what's going on once you've read through the discussion of the problem formulation and our brief comments on the Implementation. Get in touch if you need more information.
The sketch below shows the problem setup: An (inviscid) air finger propagates at a steady speed 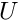 into a 2D rigid-walled, fluid-filled channel of half-width
into a 2D rigid-walled, fluid-filled channel of half-width 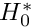 . In the process it displaces some of the viscous fluid (of density
. In the process it displaces some of the viscous fluid (of density 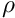 , viscosity
, viscosity 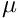 and surface tension
and surface tension  ) and deposits a film of thickness
) and deposits a film of thickness  on the channel walls. [Note that, for the sake of simplicity, we ignore the effect of transverse gravity in this discussion; the driver code listed below allows the specification of a gravitational body force which will break the up-down symmetry of the solution.]
on the channel walls. [Note that, for the sake of simplicity, we ignore the effect of transverse gravity in this discussion; the driver code listed below allows the specification of a gravitational body force which will break the up-down symmetry of the solution.]
is then determined by overall continuity.
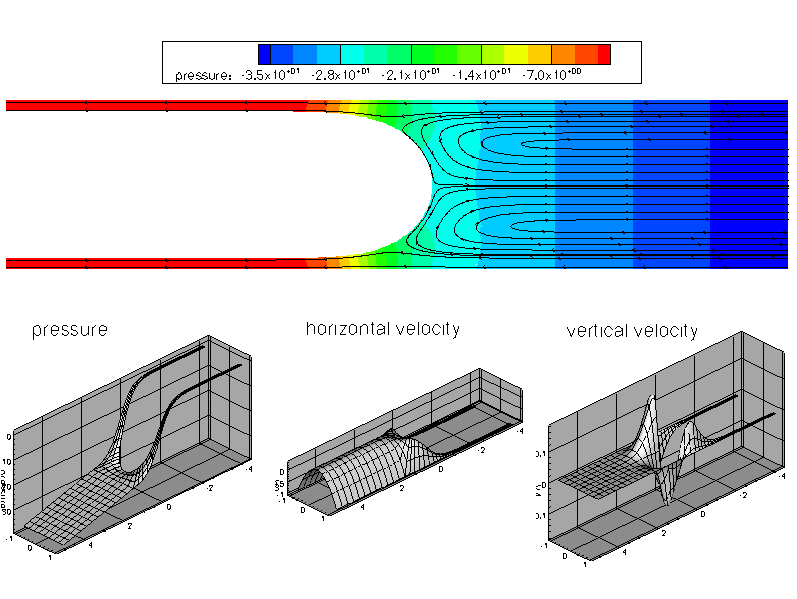
and here is a comparison of the computational predictions for the bubble pressure and film thickness against Bretherton's famous asymptotic predictions (valid only for small capillary numbers!):
The discretisation of the problem is reasonably straightforward, the most (human-)time-consuming part being the creation of the spine mesh, discussed in another tutorial. The real complication arises from the fact that the application of the "inflow condition" (1) at the right end of the domain – superficially a Dirichlet condition for the velocity – depends on the non-dimensional film thickness 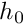 at the left end of the domain. This introduces a non-local interaction between these degrees of freedom. We handle this by providing a templated wrapper class
at the left end of the domain. This introduces a non-local interaction between these degrees of freedom. We handle this by providing a templated wrapper class BrethertonElement (templated by the type of the "wrapped" fluid element) which allows the specification of the film thickness as external Data (i.e. Data whose values affect the element's residuals but are not determined by the element; see the discussion of oomph-lib's various types of elemental Data in the "bottom up" discussion of the library's data structure). Read the driver code listed below to see how it works!
#include<algorithm>
#include "generic.h"
#include "navier_stokes.h"
#include "fluid_interface.h"
#include "meshes/bretherton_spine_mesh.h"
using namespace std;
{
void inflow(
const Vector<double>& x, Vector<double>& veloc)
{
#ifdef PARANOID
std::ostringstream error_stream;
bool throw_error=false;
{
error_stream << "You must set H_lo_pt\n";
throw_error = true;
}
{
error_stream << "You must set H_up_pt\n";
throw_error = true;
}
{
error_stream << "You must set Y_lo_pt\n";
throw_error = true;
}
{
error_stream << "You must set Y_up_pt\n";
throw_error = true;
}
if(throw_error)
{
throw OomphLibError(error_stream.str(),
OOMPH_CURRENT_FUNCTION,
OOMPH_EXCEPTION_LOCATION);
}
#endif
double C =6.0*(2.0*h_av+y_lo-y_up)/
(y_up*y_up*y_up-y_lo*y_lo*y_lo-h_av*y_up*
y_up*y_up+h_av*y_lo*y_lo*y_lo-3.0*y_lo*y_up*y_up+
3.0*y_lo*y_lo*y_up+3.0*y_lo*y_up*y_up*h_av-3.0*y_lo*y_lo*y_up*h_av);
double y=x[1];
veloc[0]=-1.0+C*(1.0-h_av)*((y_lo-y)*(y_up-y));
veloc[1]=0.0;
}
}
template<class ELEMENT>
{
public:
typedef void (*InflowFctPt)(const Vector<double>& x, Vector<double>& veloc);
void activate_inflow_dependency_on_external_data(
const Vector<Data*>& inflow_ext_data,
const unsigned& inflow_boundary,
InflowFctPt inflow_fct_pt)
{
unsigned n_ext=inflow_ext_data.size();
Inflow_ext_data.resize(n_ext);
for (unsigned i=0;i<n_ext;i++)
{
Inflow_ext_data[i]=inflow_ext_data[i];
}
Inflow_boundary=inflow_boundary;
Inflow_fct_pt=inflow_fct_pt;
}
void assign_local_eqn_numbers(const bool &store_local_dof_pt)
{
ELEMENT::assign_local_eqn_numbers(store_local_dof_pt);
unsigned local_eqn_count = this->ndof();
unsigned max_nvalue=0;
unsigned n_ext=Inflow_ext_data.size();
for (unsigned i=0;i<n_ext;i++)
{
Data* data_pt=Inflow_ext_data[i];
unsigned n_val=data_pt->nvalue();
if (n_val>max_nvalue) max_nvalue=n_val;
}
Inflow_ext_data_eqn.resize(n_ext,max_nvalue);
std::deque<unsigned long> global_eqn_number_queue;
for (unsigned i=0;i<n_ext;i++)
{
Data* data_pt=Inflow_ext_data[i];
unsigned n_val=data_pt->nvalue();
for (unsigned ival=0;ival<n_val;ival++)
{
long eqn_number=data_pt->eqn_number(ival);
if (eqn_number>=0)
{
global_eqn_number_queue.push_back(eqn_number);
Inflow_ext_data_eqn(i,ival)=local_eqn_count;
local_eqn_count++;
}
else
{
Inflow_ext_data_eqn(i,ival)=-1;
}
}
}
this->add_global_eqn_numbers(global_eqn_number_queue,
GeneralisedElement::Dof_pt_deque);
}
void get_jacobian(Vector<double>& residuals,
DenseMatrix<double>& jacobian)
{
unsigned n_ext=Inflow_ext_data.size();
ELEMENT::get_jacobian(residuals,jacobian);
if (n_ext==0) return;
Vector<double> residuals_plus(residuals.size());
double fd_step=1.0e-8;
for (unsigned i=0;i<n_ext;i++)
{
Data* data_pt=Inflow_ext_data[i];
unsigned n_val=data_pt->nvalue();
for (unsigned ival=0;ival<n_val;ival++)
{
int local_eqn=Inflow_ext_data_eqn(i,ival);
if (local_eqn>=0)
{
double *value_pt = data_pt->value_pt(ival);
double backup = *value_pt;
*value_pt += fd_step;
reassign_inflow();
unsigned n_dof = this->ndof();
for(unsigned idof=0;idof<n_dof;idof++) {residuals_plus[idof] = 0.0;}
this->get_residuals(residuals_plus);
for(unsigned idof=0;idof<n_dof;idof++)
{
jacobian(idof,local_eqn)=
(residuals_plus[idof]-residuals[idof])/fd_step;
}
*value_pt = backup;
}
}
}
reassign_inflow();
}
private:
void reassign_inflow()
{
Vector<double> x(2);
Vector<double> veloc(2);
unsigned n_nod = this->nnode();
for (unsigned j=0;j<n_nod;j++)
{
Node* nod_pt = this->node_pt(j);
if(nod_pt->is_on_boundary(Inflow_boundary))
{
#ifdef PARANOID
for (unsigned i=0;i<2;i++)
{
if (nod_pt->eqn_number(0)>=0)
{
std::ostringstream error_stream;
error_stream
<< "We're assigning a Dirichlet condition for the "
<< i << "-th "
<< "velocity, even though it is not pinned!\n"
<< "This can't be right! I'm bailing out..."
<< std::endl;
throw OomphLibError(error_stream.str(),
OOMPH_CURRENT_FUNCTION,
OOMPH_EXCEPTION_LOCATION);
}
}
#endif
x[0]=nod_pt->x(0);
x[1]=nod_pt->x(1);
Inflow_fct_pt(x,veloc);
nod_pt->set_value(0,veloc[0]);
nod_pt->set_value(1,veloc[1]);
}
}
}
Vector<Data*> Inflow_ext_data;
DenseMatrix<int> Inflow_ext_data_eqn;
unsigned Inflow_boundary;
InflowFctPt Inflow_fct_pt;
};
{
template<>
class FaceGeometry<
BrethertonElement<SpineElement<QCrouzeixRaviartElement<2> > > >:
public virtual QElement<1,3>
{
public:
FaceGeometry() : QElement<1,3>() {}
};
template<>
class FaceGeometry<
BrethertonElement<SpineElement<QTaylorHoodElement<2> > > >:
public virtual QElement<1,3>
{
public:
FaceGeometry() : QElement<1,3>() {}
};
template<>
SpineElement<QCrouzeixRaviartElement<2> > > > >: public virtual PointElement
{
public:
FaceGeometry() : PointElement() {}
};
template<>
class FaceGeometry<FaceGeometry<
BrethertonElement<SpineElement<QTaylorHoodElement<2> > > > >:
public virtual PointElement
{
public:
FaceGeometry() : PointElement() {}
};
}
template<class ELEMENT>
{
public:
void actions_before_newton_convergence_check()
{
Bulk_mesh_pt->node_update();
unsigned ibound=1;
unsigned num_nod=mesh_pt()->nboundary_node(ibound);
Vector<double> x(2);
Vector<double> veloc(2);
for (unsigned inod=0;inod<num_nod;inod++)
{
x[0]=mesh_pt()->boundary_node_pt(ibound,inod)->x(0);
x[1]=mesh_pt()->boundary_node_pt(ibound,inod)->x(1);
mesh_pt()->boundary_node_pt(ibound,inod)->set_value(0,veloc[0]);
mesh_pt()->boundary_node_pt(ibound,inod)->set_value(1,veloc[1]);
}
}
void actions_before_newton_solve() {}
void actions_after_newton_solve() {}
void fix_pressure(const unsigned &e, const unsigned &l,
const double &pvalue)
{
dynamic_cast<ELEMENT *>(Bulk_mesh_pt->element_pt(e))->
fix_pressure(l,pvalue);
}
void activate_inflow_dependency();
void parameter_study(const unsigned& nsteps);
void doc_solution(DocInfo& doc_info);
private:
ELEMENT* Control_element_pt;
ofstream Trace_file;
BrethertonSpineMesh<ELEMENT,SpineLineFluidInterfaceElement<ELEMENT> >*
Bulk_mesh_pt;
};
template<class ELEMENT>
{
unsigned nx1=24;
unsigned nx2=6;
unsigned nx3=12;
unsigned nhalf=4;
unsigned nh=3;
double h=0.1;
GeomObject* lower_wall_pt=new StraightLine(-1.0);
GeomObject* upper_wall_pt=new StraightLine( 1.0);
double xi0=-4.0;
double xi1=1.5;
double xi2=5.0;
Bulk_mesh_pt = new BrethertonSpineMesh<ELEMENT,
SpineLineFluidInterfaceElement<ELEMENT> >
(nx1,nx2,nx3,nh,nhalf,h,lower_wall_pt,upper_wall_pt,xi0,0.0,xi1,xi2);
mesh_pt()=Bulk_mesh_pt;
Control_element_pt=Bulk_mesh_pt->control_element_pt();
Bulk_mesh_pt->spine_pt(0)->spine_height_pt()->value_pt(0);
unsigned last_spine=Bulk_mesh_pt->nfree_surface_spines()-1;
Bulk_mesh_pt->spine_pt(last_spine)->spine_height_pt()->value_pt(0);
unsigned ibound=1;
Bulk_mesh_pt->boundary_node_pt(ibound,0)->x_pt(0,1);
unsigned nnod=Bulk_mesh_pt->nboundary_node(ibound);
Bulk_mesh_pt->boundary_node_pt(ibound,nnod-1)->x_pt(0,1);
activate_inflow_dependency();
for(unsigned ibound=0;ibound<=2;ibound++)
{
unsigned num_nod=mesh_pt()->nboundary_node(ibound);
for (unsigned inod=0;inod<num_nod;inod++)
{
mesh_pt()->boundary_node_pt(ibound,inod)->pin(0);
mesh_pt()->boundary_node_pt(ibound,inod)->pin(1);
}
}
for(unsigned ibound=3;ibound<=5;ibound+=2)
{
unsigned num_nod=mesh_pt()->nboundary_node(ibound);
for (unsigned inod=0;inod<num_nod;inod++)
{
mesh_pt()->boundary_node_pt(ibound,inod)->pin(0);
mesh_pt()->boundary_node_pt(ibound,inod)->pin(1);
}
}
unsigned central_spine=(Bulk_mesh_pt->nfree_surface_spines()-1)/2;
Bulk_mesh_pt->spine_pt(central_spine)->spine_height_pt()->pin(0);
for (unsigned ibound=0;ibound<=2;ibound+=2)
{
unsigned num_nod=mesh_pt()->nboundary_node(ibound);
for (unsigned inod=0;inod<num_nod;inod++)
{
mesh_pt()->boundary_node_pt(ibound,inod)->set_value(0,-1.0);
mesh_pt()->boundary_node_pt(ibound,inod)->set_value(1, 0.0);
}
}
for (unsigned ibound=3;ibound<=5;ibound+=2)
{
unsigned num_nod=mesh_pt()->nboundary_node(ibound);
for (unsigned inod=0;inod<num_nod;inod++)
{
mesh_pt()->boundary_node_pt(ibound,inod)->set_value(0,-1.0);
mesh_pt()->boundary_node_pt(ibound,inod)->set_value(1, 0.0);
}
}
unsigned n_bulk=Bulk_mesh_pt->nbulk();
for(unsigned i=0;i<n_bulk;i++)
{
ELEMENT *el_pt = dynamic_cast<ELEMENT*>(Bulk_mesh_pt->
bulk_element_pt(i));
}
unsigned interface_element_pt_range = Bulk_mesh_pt->ninterface_element();
for(unsigned i=0;i<interface_element_pt_range;i++)
{
SpineLineFluidInterfaceElement<ELEMENT>* el_pt =
dynamic_cast<SpineLineFluidInterfaceElement<ELEMENT>*>
(Bulk_mesh_pt->interface_element_pt(i));
}
Bulk_mesh_pt->node_update();
cout << "Number of unknowns: " << assign_eqn_numbers() << std::endl;
}
template<class ELEMENT>
{
Vector<Data*> outflow_spines(2);
outflow_spines[0]=Bulk_mesh_pt->spine_pt(0)->spine_height_pt();
unsigned last_spine=Bulk_mesh_pt->nfree_surface_spines()-1;
outflow_spines[1]=Bulk_mesh_pt->spine_pt(last_spine)->spine_height_pt();;
unsigned ibound=1;
unsigned nel=Bulk_mesh_pt->nboundary_element(ibound);
for (unsigned e=0;e<nel;e++)
{
ELEMENT* el_pt=dynamic_cast<ELEMENT*>(Bulk_mesh_pt->
boundary_element_pt(ibound,e));
el_pt->activate_inflow_dependency_on_external_data(
}
}
template<class ELEMENT>
{
ofstream some_file;
char filename[100];
unsigned npts=5;
Vector<double> s(2);
s[0]=1.0;
s[1]=1.0;
unsigned last_spine=Bulk_mesh_pt->nfree_surface_spines()-1;
Trace_file << " " << Bulk_mesh_pt->spine_pt(0)->height();
Trace_file << " " << Bulk_mesh_pt->spine_pt(last_spine)->height();
Trace_file << " " << -Control_element_pt->interpolated_p_nst(s)*
Trace_file << " " << Control_element_pt->interpolated_u_nst(s,0);
Trace_file << " " << Control_element_pt->interpolated_u_nst(s,1);
Trace_file << std::endl;
sprintf(filename,"%s/soln%i.dat",doc_info.directory().c_str(),
doc_info.number());
some_file.open(filename);
Bulk_mesh_pt->output(some_file,npts);
some_file.close();
sprintf(filename,"%s/boundaries%i.dat",doc_info.directory().c_str(),
doc_info.number());
some_file.open(filename);
Bulk_mesh_pt->output_boundaries(some_file);
some_file.close();
}
template<class ELEMENT>
{
Problem::Max_residuals=100.0;
DocInfo doc_info;
doc_info.set_directory("RESLT");
doc_info.number()=0;
char filename[100];
sprintf(filename,"%s/trace.dat",doc_info.directory().c_str());
Trace_file.open(filename);
Trace_file << "VARIABLES=\"Ca\",";
Trace_file << "\"h<sub>bottom</sub>\",\"h<sub>too</sub>\",";
Trace_file << "\"h<sub>Bretherton</sub>\",\"p<sub>tip</sub>\",";
Trace_file << "\"p<sub>tip (Bretherton)</sub>\",\"u<sub>stag</sub>\",";
Trace_file << "\"v<sub>stag</sub>\"";
Trace_file << "\"<greek>a</greek><sub>bottom</sub>\",";
Trace_file << "\"<greek>a</greek><sub>top</sub>\"";
Trace_file << std::endl;
double factor=2.0;
doc_solution(doc_info);
for (unsigned step=1;step<=nsteps;step++)
{
cout << std::endl << "STEP " << step << std::endl;
double maxres=100.0;
while (true)
{
cout << "Checking max. res for Ca = "
DoubleVector residuals;
actions_before_newton_solve();
actions_before_newton_convergence_check();
get_residuals(residuals);
double max_res=residuals.max();
cout << max_res;
if (max_res>maxres)
{
cout << ". Too big!" << std::endl;
factor=1.0+(factor-1.0)/1.5;
cout << "New reduction factor: " << factor << std::endl;
continue;
}
else
{
cout << ". OK" << std::endl << std::endl;
break;
}
}
cout << "Solving for capillary number: "
newton_solve();
doc_info.number()++;
doc_solution(doc_info);
}
}
int main(
int argc,
char* argv[])
{
CommandLineArgs::setup(argc,argv);
problem.self_test();
unsigned nstep;
if (CommandLineArgs::Argc>1)
{
nstep=2;
}
else
{
nstep=30;
}
}
int main(int argc, char *argv[])
Driver code for unsteady two-layer fluid problem. If there are any command line arguments,...
"Bretherton element" is a fluid element (of type ELEMENT) for which the (inflow) velocity at those no...
////////////////////////////////////////////////////////////////// //////////////////////////////////...
void activate_inflow_dependency()
Activate the dependency of the inflow velocity on the spine heights at the outflow.
BrethertonProblem()
Constructor:
void doc_solution(DocInfo &doc_info)
Doc the solution.
void parameter_study(const unsigned &nsteps)
Run a parameter study; perform specified number of steps.
Namepspace for global parameters.
double ReSt
Womersley = Reynolds times Strouhal.
void inflow(const Vector< double > &x, Vector< double > &veloc)
Set inflow velocity, based on spine heights at outflow and channel width at inflow.
double * H_lo_pt
Pointer to film thickness at outflow on the lower wall.
Vector< double > G(2)
Direction of gravity.
double * Y_up_pt
Pointer to y-position at inflow on the upper wall.
double * H_up_pt
Pointer to film thickness at outflow on the upper wall.
double * Y_lo_pt
Pointer to y-position at inflow on the lower wall.
double Ca
Capillary number.
double ReInvFr
Product of Reynolds and Froude number.
double Re
Reynolds number.
![]() into a 2D rigid-walled, fluid-filled channel of half-width
into a 2D rigid-walled, fluid-filled channel of half-width ![]() . In the process it displaces some of the viscous fluid (of density
. In the process it displaces some of the viscous fluid (of density ![]() , viscosity
, viscosity ![]() and surface tension
and surface tension ![]() ) and deposits a film of thickness
) and deposits a film of thickness ![]() on the channel walls. [Note that, for the sake of simplicity, we ignore the effect of transverse gravity in this discussion; the driver code listed below allows the specification of a gravitational body force which will break the up-down symmetry of the solution.]
on the channel walls. [Note that, for the sake of simplicity, we ignore the effect of transverse gravity in this discussion; the driver code listed below allows the specification of a gravitational body force which will break the up-down symmetry of the solution.]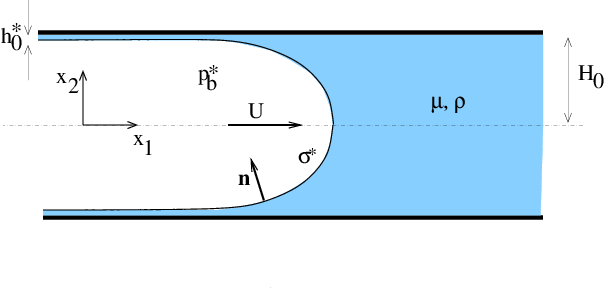
![]() , the lengths on
, the lengths on ![]() and the pressure on the viscous scale,
and the pressure on the viscous scale, ![]() and solve the problem in a frame moving with the velocity of the finger. The flow is then governed by the non-dimensional Navier-Stokes equations
and solve the problem in a frame moving with the velocity of the finger. The flow is then governed by the non-dimensional Navier-Stokes equations ![]()
![]()
![]() . On the free fluid surface, whose outer unit normal we denote by
. On the free fluid surface, whose outer unit normal we denote by ![]() , the fluid normal velocity vanishes,
, the fluid normal velocity vanishes, ![]()
![]() relative to the bubble pressure
relative to the bubble pressure ![]() . Then the dynamic boundary condition implies that
. Then the dynamic boundary condition implies that ![]()
![]() is the non-dimensional interface curvature and the capillary number
is the non-dimensional interface curvature and the capillary number ![]() is a non-dimensional measure of the bubble velocity. The no-slip condition on the channel walls requires that
is a non-dimensional measure of the bubble velocity. The no-slip condition on the channel walls requires that ![]() at
at ![]() . Far behind the bubble tip, the fluid remains stationary on the walls. In the moving frame of reference, this corresponds to the plug flow profile
. Far behind the bubble tip, the fluid remains stationary on the walls. In the moving frame of reference, this corresponds to the plug flow profile ![]() as
as ![]() . The residual film thickness
. The residual film thickness ![]() has to be determined as part of the solution and it determines the volume flux through the system. The Poiseuille velocity profile far ahead of the bubble tip,
has to be determined as part of the solution and it determines the volume flux through the system. The Poiseuille velocity profile far ahead of the bubble tip, ![]()
![]() at the left end of the domain. This introduces a non-local interaction between these degrees of freedom. We handle this by providing a templated wrapper class
at the left end of the domain. This introduces a non-local interaction between these degrees of freedom. We handle this by providing a templated wrapper class