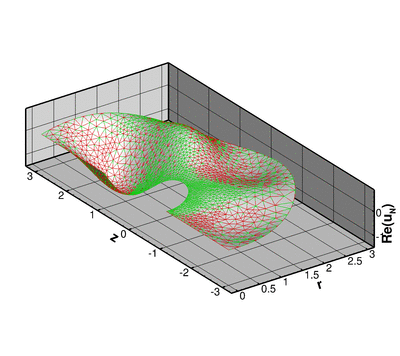
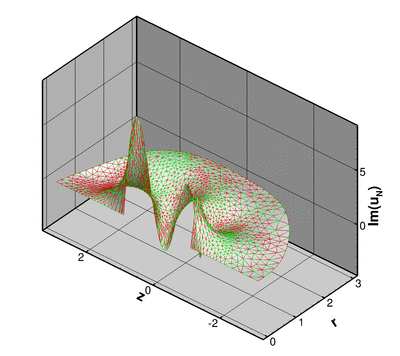
In this document we discuss the spatially-adaptive finite-element-based solution of the 3D Helmholtz equation in cylindrical polar coordinates, using a Fourier-decomposition of the solution in the azimuthal direction.
The driver code is very similar to the one discussed in another tutorial – the main purpose of the current tutorial is to demonstrate the use of spatial adaptivity on unstructured meshes.
A specific example

We will solve the azimuthally Fourier-decomposed Helmholtz equation
$\nabla^2 {u_{N}}(r,z) + \left(k^2-\frac{N^2}{r^2}\right) u_N(r,z) = 0, \ \ \ \ \ \ \ \ \ \ \ \ (1)$
where is the azimuthal wavenumber, in the finite domain $1 < \sqrt{r^2 + z^2} < 3$ . We impose the Sommerfeld radiation condition at the outer boundary of the computational domain at $\sqrt{r^2 + z^2} = 3$ , using a Dirichlet-to-Neumann mapping, and apply flux boundary condition on the surface of the unit-sphere (where $\sqrt{r^2 + z^2} = 1$ ) such that the exact solution is given by
$u_N(r,z)=u_N^{[exact]}(r,z)=\sum_{l=N}^{N_{\rm terms}} h_{l}^{(1)}(k\sqrt{r^2+z^2}) \ P_{l}^{N}\left(\frac{z}{\sqrt{r^2+z^2}}\right).$
This solution corresponds to the superposition of several outgoing waves that emerge from the unit sphere.
The two plots below show a comparison between the exact and computed solutions for $N_{\rm terms}=6$ , a Fourier wavenumber of , and a (squared) Helmholtz wavenumber of .
Plot of the exact (green) and computed (red) real parts of the solution of the Fourier-decomposed Helmholtz equation for N=1 and a wavenumber of k^2 = 10.
Plot of the exact (green) and computed (red) imaginary parts of the solution of the Fourier-decomposed Helmholtz equation for N=1 and a wavenumber of k^2 = 10.
The numerical solution

The driver code for this problem is very similar to the one discussed in another tutorial.
Running sdiff on the driver codes
demo_drivers/fourier_decomposed_helmholtz/sphere_scattering/sphere_scattering.cc
and
demo_drivers/fourier_decomposed_helmholtz/sphere_scattering/unstructured_sphere_scattering.cc
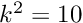shows the main differences required to discretise the computational domain with an adaptive, unstructured mesh:
The provision of the functions actions_before/after_adapt() to detach/re-attach the FaceElements that are used to enforce the Neumann boundary conditions before and after every spatial adaptation, and to pass the physical parameters to the newly created bulk elements.
The generation of an unstructured mesh whose curvilinear boundaries are represented by GeomObjects – this ensures that the domain boundaries become increasingly well resolved under mesh refinement.
That's all!
Code listing

Here's a listing of the complete driver code:
//LIC// ====================================================================
//LIC// This file forms part of oomph-lib, the object-oriented, 
//LIC// multi-physics finite-element library, available 
//LIC// at http://www.oomph-lib.org.
//LIC// 
//LIC// Copyright (C) 2006-2023 Matthias Heil and Andrew Hazel
//LIC// 
//LIC// This library is free software; you can redistribute it and/or
//LIC// modify it under the terms of the GNU Lesser General Public
//LIC// License as published by the Free Software Foundation; either
//LIC// version 2.1 of the License, or (at your option) any later version.
//LIC// 
//LIC// This library is distributed in the hope that it will be useful,
//LIC// but WITHOUT ANY WARRANTY; without even the implied warranty of
//LIC// MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE.  See the GNU
//LIC// Lesser General Public License for more details.
//LIC// 
//LIC// You should have received a copy of the GNU Lesser General Public
//LIC// License along with this library; if not, write to the Free Software
//LIC// Foundation, Inc., 51 Franklin Street, Fifth Floor, Boston, MA
//LIC// 02110-1301  USA.
//LIC// 
//LIC// The authors may be contacted at oomph-lib@maths.man.ac.uk.
//LIC// 
//LIC//====================================================================
//Driver for Fourier-decomposed  Helmholtz problem 
 
#include <complex>
#include <cmath>
 
//Generic routines
#include "generic.h"
 
// The Helmholtz equations
#include "fourier_decomposed_helmholtz.h"
 
// The mesh
#include "meshes/triangle_mesh.h"
 
// Get the Bessel functions
#include "oomph_crbond_bessel.h"
 
using namespace oomph;
using namespace std;
 
 
/// //////////////////////////////////////////////////////////////////
/// //////////////////////////////////////////////////////////////////
/// //////////////////////////////////////////////////////////////////
 
 
//===== start_of_namespace_planar_wave=================================
/// Namespace to test representation of planar wave in spherical
/// polars
//=====================================================================
namespace PlanarWave
{
 
 /// Number of terms in series
 unsigned N_terms=100;
 
 /// Wave number
 double K=3.0*MathematicalConstants::Pi;
 
 /// Imaginary unit 
 std::complex<double> I(0.0,1.0); 
 
 /// Exact solution as a Vector of size 2, containing real and imag parts
 void get_exact_u(const Vector<double>& x, Vector<double>& u)
 {
  // Switch to spherical coordinates
  double R=sqrt(x[0]*x[0]+x[1]*x[1]);
  
  double theta;
  theta=atan2(x[0],x[1]);
  
  // Argument for Bessel/Hankel functions
  double kr = K*R;  
  
  // Need half-order Bessel functions
  double bessel_offset=0.5;
 
  // Evaluate Bessel/Hankel functions
  Vector<double> jv(N_terms);
  Vector<double> yv(N_terms);
  Vector<double> djv(N_terms);
  Vector<double> dyv(N_terms);
  double order_max_in=double(N_terms-1)+bessel_offset;
  double order_max_out=0;
  
  // This function returns vectors containing 
  // J_k(x), Y_k(x) and their derivatives
  // up to k=order_max, with k increasing in
  // integer increments starting with smallest
  // positive value. So, e.g. for order_max=3.5
  // jv[0] contains J_{1/2}(x),
  // jv[1] contains J_{3/2}(x),
  // jv[2] contains J_{5/2}(x),
  // jv[3] contains J_{7/2}(x).
  CRBond_Bessel::bessjyv(order_max_in,
                         kr,
                         order_max_out,
                         &jv[0],&yv[0],
                         &djv[0],&dyv[0]);
  
  
  // Assemble  exact solution (actually no need to add terms
  // below i=N_fourier as Legendre polynomial would be zero anyway)
  complex<double> u_ex(0.0,0.0);
  for(unsigned i=0;i<N_terms;i++)
   {
    //Associated_legendre_functions
    double p=Legendre_functions_helper::plgndr2(i,0,cos(theta));
    
    // Set exact solution
    u_ex+=(2.0*i+1.0)*pow(I,i)*
     sqrt(MathematicalConstants::Pi/(2.0*kr))*jv[i]*p;
   }
  
  // Get the real & imaginary part of the result
  u[0]=u_ex.real();
  u[1]=u_ex.imag();
  
 }//end of get_exact_u
 
 
 /// Plot 
 void plot()
 {
  unsigned nr=20;
  unsigned nz=100;
  unsigned nt=40;
 
  ofstream some_file("planar_wave.dat");
 
  for (unsigned i_t=0;i_t<nt;i_t++)
   {
    double t=2.0*MathematicalConstants::Pi*double(i_t)/double(nt-1);
 
    some_file << "ZONE I="<< nz << ", J="<< nr << std::endl;
    
    Vector<double> x(2);
    Vector<double> u(2);
    for (unsigned i=0;i<nr;i++)
     {
      x[0]=0.001+double(i)/double(nr-1);
      for (unsigned j=0;j<nz;j++)
       {
        x[1]=double(j)/double(nz-1);
        get_exact_u(x,u); 
        complex<double> uu=complex<double>(u[0],u[1])*exp(-I*t);
        some_file << x[0] << " " << x[1] << " " 
                  << uu.real() << " " << uu.imag() << "\n";
       }
     } 
   }
 }
 
}
 
 
/// //////////////////////////////////////////////////////////////////
/// //////////////////////////////////////////////////////////////////
/// //////////////////////////////////////////////////////////////////
 
 
//===== start_of_namespace=============================================
/// Namespace for the Fourier decomposed Helmholtz problem parameters
//=====================================================================
namespace ProblemParameters
{
 /// Square of the wavenumber
 double K_squared=10.0;
 
 /// Fourier wave number
 int N_fourier=3;
 
 /// Number of terms in computation of DtN boundary condition
 unsigned Nterms_for_DtN=6;
 
 /// Number of terms in the exact solution
 unsigned N_terms=6; 
 
 /// Coefficients in the exact solution
 Vector<double> Coeff(N_terms,1.0);
 
 /// Imaginary unit 
 std::complex<double> I(0.0,1.0); 
 
 /// Exact solution as a Vector of size 2, containing real and imag parts
 void get_exact_u(const Vector<double>& x, Vector<double>& u)
 {
  // Switch to spherical coordinates
  double R=sqrt(x[0]*x[0]+x[1]*x[1]);
  
  double theta;
  theta=atan2(x[0],x[1]);
  
  // Argument for Bessel/Hankel functions
  double kr = sqrt(K_squared)*R;  
  
  // Need half-order Bessel functions
  double bessel_offset=0.5;
 
  // Evaluate Bessel/Hankel functions
  Vector<double> jv(N_terms);
  Vector<double> yv(N_terms);
  Vector<double> djv(N_terms);
  Vector<double> dyv(N_terms);
  double order_max_in=double(N_terms-1)+bessel_offset;
  double order_max_out=0;
  
  // This function returns vectors containing 
  // J_k(x), Y_k(x) and their derivatives
  // up to k=order_max, with k increasing in
  // integer increments starting with smallest
  // positive value. So, e.g. for order_max=3.5
  // jv[0] contains J_{1/2}(x),
  // jv[1] contains J_{3/2}(x),
  // jv[2] contains J_{5/2}(x),
  // jv[3] contains J_{7/2}(x).
  CRBond_Bessel::bessjyv(order_max_in,
                         kr,
                         order_max_out,
                         &jv[0],&yv[0],
                         &djv[0],&dyv[0]);
  
  
  // Assemble  exact solution (actually no need to add terms
  // below i=N_fourier as Legendre polynomial would be zero anyway)
  complex<double> u_ex(0.0,0.0);
  for(unsigned i=N_fourier;i<N_terms;i++)
   {
    //Associated_legendre_functions
    double p=Legendre_functions_helper::plgndr2(i,N_fourier,
                                                cos(theta));
    // Set exact solution
    u_ex+=Coeff[i]*sqrt(MathematicalConstants::Pi/(2.0*kr))*(jv[i]+I*yv[i])*p;
   }
  
  // Get the real & imaginary part of the result
  u[0]=u_ex.real();
  u[1]=u_ex.imag();
  
 }//end of get_exact_u
 
 
 /// Get -du/dr (spherical r) for exact solution. Equal to prescribed
 /// flux on inner boundary.
 void exact_minus_dudr(const Vector<double>& x, std::complex<double>& flux)
 {
  // Initialise flux
  flux=std::complex<double>(0.0,0.0);
  
  // Switch to spherical coordinates
  double R=sqrt(x[0]*x[0]+x[1]*x[1]);
  
  double theta;
  theta=atan2(x[0],x[1]);
  
  // Argument for Bessel/Hankel functions
  double kr=sqrt(K_squared)*R;  
 
  // Helmholtz wavenumber
  double k=sqrt(K_squared);
 
  // Need half-order Bessel functions
  double bessel_offset=0.5;
 
  // Evaluate Bessel/Hankel functions
  Vector<double> jv(N_terms);
  Vector<double> yv(N_terms);
  Vector<double> djv(N_terms);
  Vector<double> dyv(N_terms);
  double order_max_in=double(N_terms-1)+bessel_offset;
  double order_max_out=0;
  
  // This function returns vectors containing 
  // J_k(x), Y_k(x) and their derivatives
  // up to k=order_max, with k increasing in
  // integer increments starting with smallest
  // positive value. So, e.g. for order_max=3.5
  // jv[0] contains J_{1/2}(x),
  // jv[1] contains J_{3/2}(x),
  // jv[2] contains J_{5/2}(x),
  // jv[3] contains J_{7/2}(x).
  CRBond_Bessel::bessjyv(order_max_in,
                         kr,
                         order_max_out,
                         &jv[0],&yv[0],
                         &djv[0],&dyv[0]);
  
  
  // Assemble  exact solution (actually no need to add terms
  // below i=N_fourier as Legendre polynomial would be zero anyway)
  complex<double> u_ex(0.0,0.0);
  for(unsigned i=N_fourier;i<N_terms;i++)
   {
    //Associated_legendre_functions
    double p=Legendre_functions_helper::plgndr2(i,N_fourier,
                                                cos(theta));
    // Set flux of exact solution
    flux-=Coeff[i]*sqrt(MathematicalConstants::Pi/(2.0*kr))*p*
     ( k*(djv[i]+I*dyv[i]) - (0.5*(jv[i]+I*yv[i])/R) );
   }
  
 }// end of exact_normal_derivative
 
 
 
} // end of namespace
 
 
 
/// //////////////////////////////////////////////////////////////////
/// //////////////////////////////////////////////////////////////////
/// //////////////////////////////////////////////////////////////////
 
 
//========= start_of_problem_class=====================================
/// Problem class 
//=====================================================================
template<class ELEMENT> 
class FourierDecomposedHelmholtzProblem : public Problem
{
 
public:
 
 /// Constructor
 FourierDecomposedHelmholtzProblem();
 
 /// Destructor (empty)
 ~FourierDecomposedHelmholtzProblem(){}
 
 /// Update the problem specs before solve (empty)
 void actions_before_newton_solve(){}
 
 /// Update the problem after solve (empty)
 void actions_after_newton_solve(){}
 
 /// Doc the solution. DocInfo object stores flags/labels for where the
 /// output gets written to
 void doc_solution(DocInfo& doc_info);
  
 /// Recompute gamma integral before checking Newton residuals
 void actions_before_newton_convergence_check()
  {
   if (!CommandLineArgs::command_line_flag_has_been_set("--square_domain"))
    {
     Helmholtz_outer_boundary_mesh_pt->setup_gamma();
    }
  }
 
 /// Actions before adapt: Wipe the mesh of prescribed flux elements
 void actions_before_adapt();
 
 /// Actions after adapt: Rebuild the mesh of prescribed flux elements
 void actions_after_adapt();
 
 /// Check gamma computation
 void check_gamma(DocInfo& doc_info);
  
private:
 
 /// Create BC elements on outer boundary
 void create_outer_bc_elements();
 
 /// Create flux elements on inner boundary
 void create_flux_elements_on_inner_boundary();
 
 
 /// Delete boundary face elements and wipe the surface mesh
 void delete_face_elements( Mesh* const & boundary_mesh_pt)
  {
   // Loop over the surface elements
   unsigned n_element = boundary_mesh_pt->nelement();
   for(unsigned e=0;e<n_element;e++)
    {
     // Kill surface element
     delete  boundary_mesh_pt->element_pt(e);
    }
   
   // Wipe the mesh
   boundary_mesh_pt->flush_element_and_node_storage();
   
  } 
 
#ifdef ADAPTIVE
 
 /// Pointer to the "bulk" mesh
 RefineableTriangleMesh<ELEMENT>* Bulk_mesh_pt;
 
#else
 
 /// Pointer to the "bulk" mesh
 TriangleMesh<ELEMENT>* Bulk_mesh_pt;
 
#endif
  
 /// Pointer to mesh containing the DtN boundary
 /// condition elements
 FourierDecomposedHelmholtzDtNMesh<ELEMENT>* Helmholtz_outer_boundary_mesh_pt;
 
 /// on the inner boundary
 Mesh* Helmholtz_inner_boundary_mesh_pt;
 
 /// Trace file
 ofstream Trace_file;
 
}; // end of problem class
 
 
 
//=====================start_of_actions_before_adapt======================
/// Actions before adapt: Wipe the mesh of face elements
//========================================================================
template<class ELEMENT>
void FourierDecomposedHelmholtzProblem<ELEMENT>::actions_before_adapt()
{ 
 // Kill the flux elements and wipe the boundary meshs
 if (!CommandLineArgs::command_line_flag_has_been_set("--square_domain"))
  {
   delete_face_elements(Helmholtz_outer_boundary_mesh_pt);
  }
 delete_face_elements(Helmholtz_inner_boundary_mesh_pt);
 
 // Rebuild the Problem's global mesh from its various sub-meshes
 rebuild_global_mesh();
 
}// end of actions_before_adapt
 
 
//=====================start_of_actions_after_adapt=======================
///  Actions after adapt: Rebuild the face element meshes
//========================================================================
template<class ELEMENT>
void FourierDecomposedHelmholtzProblem<ELEMENT>::actions_after_adapt()
{
 
 
 // Complete the build of all elements so they are fully functional
 
 // Loop over the Helmholtz bulk elements to set up element-specific 
 // things that cannot be handled by constructor: Pass pointer to 
 // wave number squared
 unsigned n_element = Bulk_mesh_pt->nelement();
 for(unsigned e=0;e<n_element;e++)
  {
   // Upcast from GeneralisedElement to Helmholtz bulk element
   ELEMENT *el_pt = dynamic_cast<ELEMENT*>(Bulk_mesh_pt->element_pt(e));
   
   //Set the k_squared  pointer
   el_pt->k_squared_pt() = &ProblemParameters::K_squared;
 
   // Set pointer to Fourier wave number
   el_pt->fourier_wavenumber_pt()=&ProblemParameters::N_fourier;
  }
 
 // Create prescribed-flux elements and BC elements 
 // from all elements that are adjacent to the boundaries and add them to 
 // Helmholtz_boundary_meshes
 create_flux_elements_on_inner_boundary();
 if (!CommandLineArgs::command_line_flag_has_been_set("--square_domain"))
  {
   create_outer_bc_elements();
  }
 
 // Rebuild the Problem's global mesh from its various sub-meshes
 rebuild_global_mesh();
  
}// end of actions_after_adapt
 
 
//=======start_of_constructor=============================================
/// Constructor for Fourier-decomposed Helmholtz problem
//========================================================================
template<class ELEMENT>
FourierDecomposedHelmholtzProblem<ELEMENT>::
FourierDecomposedHelmholtzProblem()
{ 
 
 // Open trace file
 Trace_file.open("RESLT/trace.dat");
 
 // Create circles representing inner and outer boundary
 double x_c=0.0;
 double y_c=0.0;
 double r_min=1.0;
 double r_max=3.0;
 Circle* inner_circle_pt=new Circle(x_c,y_c,r_min);
 Circle* outer_circle_pt=new Circle(x_c,y_c,r_max);
 
 // Edges/boundary segments making up outer boundary
 //-------------------------------------------------
 Vector<TriangleMeshCurveSection*> outer_boundary_line_pt(4);
 
 // Number of segments used for representing the curvilinear boundaries
 unsigned n_segments = 20;
 
 // All poly boundaries are defined by two vertices
 Vector<Vector<double> > boundary_vertices(2);
 
 
 // Bottom straight boundary on symmetry line
 //------------------------------------------
 boundary_vertices[0].resize(2);
 boundary_vertices[0][0]=0.0;
 boundary_vertices[0][1]=-r_min;
 boundary_vertices[1].resize(2);
 boundary_vertices[1][0]=0.0;
 boundary_vertices[1][1]=-r_max;
 
 unsigned boundary_id=0;
 outer_boundary_line_pt[0]=
  new TriangleMeshPolyLine(boundary_vertices,boundary_id);
 
 
 if (CommandLineArgs::command_line_flag_has_been_set("--square_domain"))
  {
   // Square outer boundary:
   //-----------------------
 
   Vector<Vector<double> > boundary_vertices(4);
   boundary_vertices[0].resize(2);
   boundary_vertices[0][0]=0.0;
   boundary_vertices[0][1]=-r_max;
   boundary_vertices[1].resize(2);
   boundary_vertices[1][0]=r_max;
   boundary_vertices[1][1]=-r_max;
   boundary_vertices[2].resize(2);
   boundary_vertices[2][0]=r_max;
   boundary_vertices[2][1]=r_max;
   boundary_vertices[3].resize(2);
   boundary_vertices[3][0]=0.0;
   boundary_vertices[3][1]=r_max;
 
   boundary_id=1;
   outer_boundary_line_pt[1]=
    new TriangleMeshPolyLine(boundary_vertices,boundary_id);
  }
 else
  {
   // Outer circular boundary:
   //-------------------------
   // The intrinsic coordinates for the beginning and end of the curve
   double s_start = -0.5*MathematicalConstants::Pi;
   double s_end   =  0.5*MathematicalConstants::Pi;
   
   boundary_id = 1;
   outer_boundary_line_pt[1]=
    new TriangleMeshCurviLine(outer_circle_pt,
                              s_start,
                              s_end,
                              n_segments,
                              boundary_id);
  }
 
 
 // Top straight boundary on symmetry line
 //---------------------------------------
 boundary_vertices[0][0]=0.0;
 boundary_vertices[0][1]=r_max;
 boundary_vertices[1][0]=0.0;
 boundary_vertices[1][1]=r_min;
 
 boundary_id=2;
 outer_boundary_line_pt[2]=
  new TriangleMeshPolyLine(boundary_vertices,boundary_id);
 
 
 // Inner circular boundary:
 //-------------------------
 
 // The intrinsic coordinates for the beginning and end of the curve
 double s_start =  0.5*MathematicalConstants::Pi;
 double s_end   =  -0.5*MathematicalConstants::Pi;
 
 boundary_id = 3;
 outer_boundary_line_pt[3]=
  new TriangleMeshCurviLine(inner_circle_pt,
                            s_start,
                            s_end,
                            n_segments,
                            boundary_id);
 
 
 // Create closed curve that defines outer boundary
 //------------------------------------------------
 TriangleMeshClosedCurve *outer_boundary_pt =
  new TriangleMeshClosedCurve(outer_boundary_line_pt);
 
 
 // Use the TriangleMeshParameters object for helping on the manage of the
 // TriangleMesh parameters. The only parameter that needs to take is the
 // outer boundary.
 TriangleMeshParameters triangle_mesh_parameters(outer_boundary_pt);
 
 // Specify maximum element area
 double element_area = 0.1; 
 triangle_mesh_parameters.element_area() = element_area;
 
#ifdef ADAPTIVE
 
 // Build "bulk" mesh
 Bulk_mesh_pt=new RefineableTriangleMesh<ELEMENT>(triangle_mesh_parameters);
 
 // Create/set error estimator
 Bulk_mesh_pt->spatial_error_estimator_pt()=new Z2ErrorEstimator;
 
 // Choose error tolerances to force some uniform refinement
 Bulk_mesh_pt->min_permitted_error()=0.00004;
 Bulk_mesh_pt->max_permitted_error()=0.0001;
 
#else
 
 // Pass the TriangleMeshParameters object to the TriangleMesh one
 Bulk_mesh_pt= new TriangleMesh<ELEMENT>(triangle_mesh_parameters);
 
#endif
 
 // Check what we've built so far...
 Bulk_mesh_pt->output("mesh.dat");
 Bulk_mesh_pt->output_boundaries("boundaries.dat");
 
 
 if (!CommandLineArgs::command_line_flag_has_been_set("--square_domain"))
  {
   // Create mesh for DtN elements on outer boundary
   Helmholtz_outer_boundary_mesh_pt=
    new FourierDecomposedHelmholtzDtNMesh<ELEMENT>(
     r_max,ProblemParameters::Nterms_for_DtN);
   
   // Populate it with elements
   create_outer_bc_elements();
  }
 
 // Create flux elements on inner boundary
 Helmholtz_inner_boundary_mesh_pt=new Mesh;
 create_flux_elements_on_inner_boundary();
 
 // Add the several sub meshes to the problem
 add_sub_mesh(Bulk_mesh_pt); 
 add_sub_mesh(Helmholtz_inner_boundary_mesh_pt); 
 if (!CommandLineArgs::command_line_flag_has_been_set("--square_domain"))
  {
   add_sub_mesh(Helmholtz_outer_boundary_mesh_pt); 
  }
 
 // Build the Problem's global mesh from its various sub-meshes
 build_global_mesh();
 
 // Complete the build of all elements so they are fully functional
 unsigned n_element = Bulk_mesh_pt->nelement();
 for(unsigned i=0;i<n_element;i++)
  {
   // Upcast from GeneralsedElement to the present element
   ELEMENT *el_pt = dynamic_cast<ELEMENT*>(Bulk_mesh_pt->element_pt(i));
   
   //Set the k_squared pointer
   el_pt->k_squared_pt()=&ProblemParameters::K_squared;
   
   // Set pointer to Fourier wave number
   el_pt->fourier_wavenumber_pt()=&ProblemParameters::N_fourier;
  }
 
 // Setup equation numbering scheme
 cout <<"Number of equations: " << assign_eqn_numbers() << std::endl; 
 
} // end of constructor
 
 
 
//=================================start_of_check_gamma===================
/// Check gamma computation: \f$ \gamma = -du/dn \f$
//========================================================================
template<class ELEMENT>
void FourierDecomposedHelmholtzProblem<ELEMENT>::check_gamma(DocInfo& doc_info)
{
 
 // Compute gamma stuff
 Helmholtz_outer_boundary_mesh_pt->setup_gamma();
 
 ofstream some_file;
 char filename[100];
 
 sprintf(filename,"%s/gamma_test%i.dat",doc_info.directory().c_str(),
         doc_info.number());
 some_file.open(filename);
  
 //first loop over elements e
 unsigned nel=Helmholtz_outer_boundary_mesh_pt->nelement();
 for (unsigned e=0;e<nel;e++)
  {
   // Get a pointer to element
   FourierDecomposedHelmholtzDtNBoundaryElement<ELEMENT>* el_pt=
    dynamic_cast<FourierDecomposedHelmholtzDtNBoundaryElement<ELEMENT>*>
    (Helmholtz_outer_boundary_mesh_pt->element_pt(e));
   
   //Set the value of n_intpt
   const unsigned n_intpt =el_pt->integral_pt()->nweight();
   
   // Get gamma at all gauss points in element
   Vector<std::complex<double> > gamma(
    Helmholtz_outer_boundary_mesh_pt->gamma_at_gauss_point(el_pt));
   
   //Loop over the integration points
   for(unsigned ipt=0;ipt<n_intpt;ipt++)
    {
     //Allocate and initialise coordiante
     Vector<double> x(el_pt->dim()+1,0.0);
     
     //Set the Vector to hold local coordinates
     unsigned n=el_pt->dim();
     Vector<double> s(n,0.0);
     for(unsigned i=0;i<n;i++)
      {
       s[i]=el_pt->integral_pt()->knot(ipt,i);
      }
     
     //Get the coordinates of the integration point
     el_pt->interpolated_x(s,x);
     
     complex<double> flux;
     ProblemParameters::exact_minus_dudr(x,flux);
     some_file << atan2(x[0],x[1]) << " " 
               << gamma[ipt].real() << " "
               << gamma[ipt].imag() << " "
               << flux.real() << " " 
               << flux.imag() << " " 
               << std::endl;
     
    }// end of loop over integration points
   
  }// end of loop over elements
 
 some_file.close();
  
}//end of output_gamma
 
 
//===============start_of_doc=============================================
/// Doc the solution: doc_info contains labels/output directory etc.
//========================================================================
template<class ELEMENT>
void FourierDecomposedHelmholtzProblem<ELEMENT>::doc_solution(DocInfo& doc_info)
{ 
 
 ofstream some_file;
 char filename[100];
 
 // Number of plot points: npts x npts
 unsigned npts=5;
  
 // Output solution 
 //-----------------
 sprintf(filename,"%s/soln%i.dat",doc_info.directory().c_str(),
         doc_info.number());
 some_file.open(filename);
 Bulk_mesh_pt->output(some_file,npts);
 some_file.close();
 
 
 // Output exact solution 
 //----------------------
 sprintf(filename,"%s/exact_soln%i.dat",doc_info.directory().c_str(),
         doc_info.number());
 some_file.open(filename);
 Bulk_mesh_pt->output_fct(some_file,npts,ProblemParameters::get_exact_u); 
 some_file.close();
 
 
 // Doc error and return of the square of the L2 error
 //---------------------------------------------------
 double error,norm;
 sprintf(filename,"%s/error%i.dat",doc_info.directory().c_str(),
         doc_info.number());
 some_file.open(filename);
 Bulk_mesh_pt->compute_error(some_file,ProblemParameters::get_exact_u,
                             error,norm); 
 some_file.close();
 
 // Doc L2 error and norm of solution
 cout << "\nNorm of error   : " << sqrt(error) << std::endl; 
 cout << "Norm of solution: " << sqrt(norm) << std::endl << std::endl;
 
 
 // Write norm of solution to trace file
 Bulk_mesh_pt->compute_norm(norm); 
 Trace_file  << norm << std::endl;
 
 
 if (!CommandLineArgs::command_line_flag_has_been_set("--square_domain"))
  {
   // Check gamma computation
   check_gamma(doc_info);
  }
 
} // end of doc
 
 
 
//============start_of_create_outer_bc_elements==============================
/// Create BC elements on outer boundary
//========================================================================
template<class ELEMENT>
void FourierDecomposedHelmholtzProblem<ELEMENT>::create_outer_bc_elements()
{
 
 // Outer boundary is boundary 1:
 unsigned b=1;
 
 // Loop over the bulk elements adjacent to boundary b?
 unsigned n_element = Bulk_mesh_pt->nboundary_element(b);
 for(unsigned e=0;e<n_element;e++)
  {
   // Get pointer to the bulk element that is adjacent to boundary b
   ELEMENT* bulk_elem_pt = dynamic_cast<ELEMENT*>(
    Bulk_mesh_pt->boundary_element_pt(b,e));
   
   //Find the index of the face of element e along boundary b 
   int face_index = Bulk_mesh_pt->face_index_at_boundary(b,e);
   
   // Build the corresponding DtN element
   FourierDecomposedHelmholtzDtNBoundaryElement<ELEMENT>* flux_element_pt = new 
    FourierDecomposedHelmholtzDtNBoundaryElement<ELEMENT>(bulk_elem_pt,
                                                          face_index);
   
   //Add the flux boundary element to the  helmholtz_outer_boundary_mesh
   Helmholtz_outer_boundary_mesh_pt->add_element_pt(flux_element_pt);
 
   // Set pointer to the mesh that contains all the boundary condition
   // elements on this boundary
   flux_element_pt->
    set_outer_boundary_mesh_pt(Helmholtz_outer_boundary_mesh_pt);
  }
 
} // end of create_outer_bc_elements
 
 
 
//============start_of_create_flux_elements=================
/// Create flux elements on inner boundary
//==========================================================
template<class ELEMENT>
void  FourierDecomposedHelmholtzProblem<ELEMENT>::
create_flux_elements_on_inner_boundary()
{
 // Apply flux bc on inner boundary (boundary 3)
 unsigned b=3;
 
// Loop over the bulk elements adjacent to boundary b
 unsigned n_element = Bulk_mesh_pt->nboundary_element(b);
 for(unsigned e=0;e<n_element;e++)
  {
   // Get pointer to the bulk element that is adjacent to boundary b
   ELEMENT* bulk_elem_pt = dynamic_cast<ELEMENT*>(
    Bulk_mesh_pt->boundary_element_pt(b,e));
   
   //Find the index of the face of element e along boundary b 
   int face_index = Bulk_mesh_pt->face_index_at_boundary(b,e);
   
   // Build the corresponding prescribed incoming-flux element
   FourierDecomposedHelmholtzFluxElement<ELEMENT>* flux_element_pt = new 
    FourierDecomposedHelmholtzFluxElement<ELEMENT>(bulk_elem_pt,face_index);
   
   //Add the prescribed incoming-flux element to the surface mesh
   Helmholtz_inner_boundary_mesh_pt->add_element_pt(flux_element_pt);
   
   // Set the pointer to the prescribed flux function
   flux_element_pt->flux_fct_pt() = &ProblemParameters::exact_minus_dudr;
 
  } //end of loop over bulk elements adjacent to boundary b
 
} // end of create flux elements on inner boundary
 
 
 
//===== start_of_main=====================================================
/// Driver code for Fourier decomposed Helmholtz problem
//========================================================================
int main(int argc, char **argv)
{
 // Store command line arguments
 CommandLineArgs::setup(argc,argv);
 
 // Define possible command line arguments and parse the ones that
 // were actually specified
 
 // Square domain without DtN
 CommandLineArgs::specify_command_line_flag("--square_domain");
 
 // Parse command line
 CommandLineArgs::parse_and_assign(); 
 
 // Doc what has actually been specified on the command line
 CommandLineArgs::doc_specified_flags();
 
 // Check if the claimed representation of a planar wave in
 // the tutorial is correct -- of course it is!
 //PlanarWave::plot();
 
 // Test Bessel/Hankel functions
 //-----------------------------
 {
  // Number of Bessel functions to be computed
  unsigned n=3;
  
  // Offset of Bessel function order (less than 1!)
  double bessel_offset=0.5;
  
  ofstream bessely_file("besselY.dat");
  ofstream bessely_deriv_file("dbesselY.dat");
  
  ofstream besselj_file("besselJ.dat");
  ofstream besselj_deriv_file("dbesselJ.dat");
  
  // Evaluate Bessel/Hankel functions
  Vector<double> jv(n+1);
  Vector<double> yv(n+1);
  Vector<double> djv(n+1);
  Vector<double> dyv(n+1);
  double x_min=0.5;
  double x_max=5.0;
  unsigned nplot=100;
  for (unsigned i=0;i<nplot;i++)
   {
    double x=x_min+(x_max-x_min)*double(i)/double(nplot-1);
    double order_max_in=double(n)+bessel_offset;
    double order_max_out=0;
    
    // This function returns vectors containing 
    // J_k(x), Y_k(x) and their derivatives
    // up to k=order_max, with k increasing in
    // integer increments starting with smallest
    // positive value. So, e.g. for order_max=3.5
    // jv[0] contains J_{1/2}(x),
    // jv[1] contains J_{3/2}(x),
    // jv[2] contains J_{5/2}(x),
    // jv[3] contains J_{7/2}(x).
    CRBond_Bessel::bessjyv(order_max_in,x,
                           order_max_out,
                           &jv[0],&yv[0],
                           &djv[0],&dyv[0]);
    bessely_file << x << " ";
    for (unsigned j=0;j<=n;j++)
     {
      bessely_file << yv[j] << " ";
     }
    bessely_file << std::endl;
    
    besselj_file << x << " ";
    for (unsigned j=0;j<=n;j++)
     {
      besselj_file << jv[j] << " ";
     }
    besselj_file << std::endl;
    
    bessely_deriv_file << x << " ";
    for (unsigned j=0;j<=n;j++)
     {
      bessely_deriv_file << dyv[j] << " ";
     }
    bessely_deriv_file << std::endl;
    
    besselj_deriv_file << x << " ";
    for (unsigned j=0;j<=n;j++)
     {
      besselj_deriv_file << djv[j] << " ";
     }
    besselj_deriv_file << std::endl;
    
   }
  bessely_file.close();
  besselj_file.close();
  bessely_deriv_file.close();
  besselj_deriv_file.close();
 }
 
 
 // Test Legrendre Polynomials
 //---------------------------
 {
  // Number of lower indices
  unsigned n=3;
    
  ofstream some_file("legendre3.dat");
  unsigned nplot=100;
  for (unsigned i=0;i<nplot;i++)
   {
    double x=double(i)/double(nplot-1);
 
    some_file << x << " ";
    for (unsigned j=0;j<=n;j++)
     {
      some_file <<  Legendre_functions_helper::plgndr2(n,j,x) << " ";
     }
    some_file << std::endl;
   }
  some_file.close();
 }
 
 
#ifdef ADAPTIVE
 
 // Create the problem with 2D six-node elements from the
 // TFourierDecomposedHelmholtzElement family. 
 FourierDecomposedHelmholtzProblem<ProjectableFourierDecomposedHelmholtzElement<
  TFourierDecomposedHelmholtzElement<3> > > problem;
 
#else
 
 // Create the problem with 2D six-node elements from the
 // TFourierDecomposedHelmholtzElement family. 
 FourierDecomposedHelmholtzProblem<TFourierDecomposedHelmholtzElement<3> > 
  problem;
 
#endif
 
 // Create label for output
 DocInfo doc_info;
 
 // Set output directory
 doc_info.set_directory("RESLT");
 
 // Solve for a few Fourier wavenumbers
 for (ProblemParameters::N_fourier=0;ProblemParameters::N_fourier<4;
      ProblemParameters::N_fourier++)
  {
   // Step number
   doc_info.number()=ProblemParameters::N_fourier;
   
 
 
#ifdef ADAPTIVE
 
 // Max. number of adaptations
 unsigned max_adapt=1;
 
   // Solve the problem with Newton's method, allowing
   // up to max_adapt mesh adaptations after every solve.
   problem.newton_solve(max_adapt);
 
#else
 
   // Solve the problem
   problem.newton_solve();
 
#endif
   
   //Output the solution
   problem.doc_solution(doc_info);
  }
 
} //end of main
 
 
 
 
 
 
 
Source files for this tutorial

The source files for this tutorial are located in the directory:

demo_drivers/fourier_decomposed_helmholtz/sphere_scattering/
The driver code is:

demo_drivers/fourier_decomposed_helmholtz/sphere_scattering/unstructured_sphere_scattering.cc
PDF file

A pdf version of this document is available.